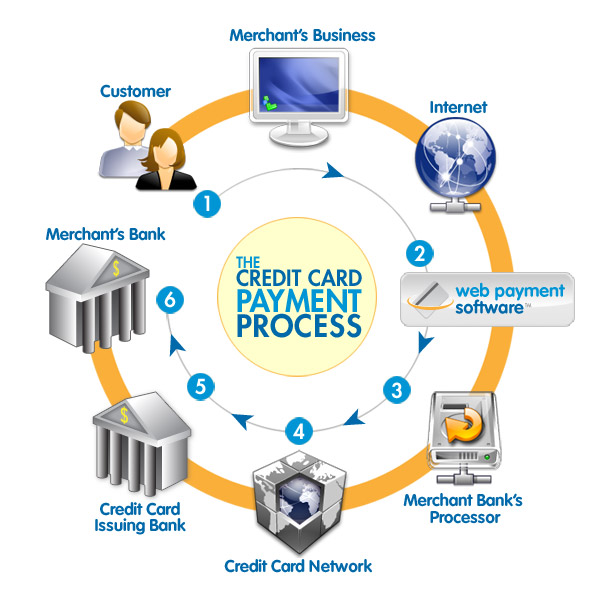
the electronic payment grid cartel
is nailing the petty merchants and service providers
to their clever incentive plan ceiling rates



1. Anything identified as a state in the standard historical atlases[13] was assumed to be a t-nation except for the Holy Roman Empire after 911 and France from 987 until 1400. In these two cases, the component states or major feudatories were considered t-nations for taxes on trade.[14] Zollvereinen were also considered t-nations for taxes on trade.
2. Where more than one state was under a single ruler, the group of states was considered a single t-nation (this includes states under the church).
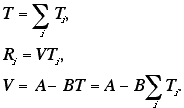
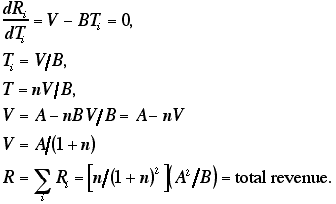

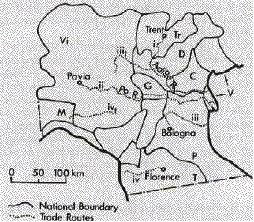
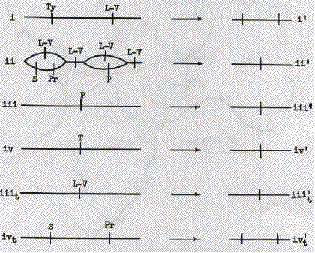 Fig. 2A.--Schematic diagrams of trade routes, northern
Italy, 1854
Fig. 2A.--Schematic diagrams of trade routes, northern
Italy, 1854
 Fig. 2B--Schematic diagrams of trade routes, northern
Italy, 1378
Fig. 2B--Schematic diagrams of trade routes, northern
Italy, 1378
| TABLE 1 | ||||||
|---|---|---|---|---|---|---|
| Route | ||||||
| i | ||||||
| ii | ||||||
| iii | ||||||
| iv | ||||||
| iiit | ||||||
| ivt | ||||||
| TABLE 2 | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
1. Cities
|
||||||||||||||
2. Cities/cities displaced
|
||||||||||||||
3. High-trade rivers
|
||||||||||||||
4. High trade/low trade
|
||||||||||||||
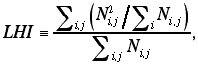
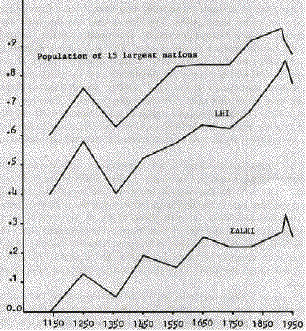 Fig. 3
Fig. 3
| TABLE 3 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
1. LHI
|
|||||||||||
2. Population of 15 largest
nations*
|
|||||||||||
3.
|
|||||||||||
4.
|
|||||||||||
| Table 4 | |||||
|---|---|---|---|---|---|
1950 Iron curtain |
1950 Non-Iron Curtain* |
1922 "Iron Curtain"** |
1922 "Non-Iron Curtain * *** | ||
| 1 | 2 | ||||
| LHI | .81 | .88 | .78 | .81 | .81 |
| Population§ | .38 | .38 | .32 | .32 | .32 |
[T]he term "Lucas critique" has survived, long after [the] original context has disappeared. It has a life of its own and means different things to different people. Sometimes it is used like a cross you are supposed to use to hold off vampires: Just waving it it an opponent defeats him. Too much of this, no matter what side you are on, becomes just name calling.
Virtually all macroeconomic models today are dynamic, stochastic and general equilibrium (where here "general equilibrium" means you have the same number of equations and unknowns)...If we narrow the definition of DSGE by using "general equilibrium" to refer to competitive or Nash equilibria where the strategy sets of each agent are made explicit in an internally consistent way then we have Kydland-Prescott and other RBC descendants and not much else.
I was [initially] convinced by Friedman and Schwartz that the 1929-33 down turn was induced by monetary factors...I concluded that a good starting point for theory would be the working hypothesis that all depressions are mainly monetary in origin. Ed Prescott was skeptical about this strategy from the beginning...
I now believe that the evidence on post-war recessions (up to but not including the one we are now in) overwhelmingly supports the dominant importance of real shocks. But I remain convinced of the importance of financial shocks in the 1930s and the years after 2008. Of course, this means I have to renounce the view that business cycles are all alike!
If the Depression continues, in some respects, to defy explanation by existing economic analysis (as I believe it does), perhaps it is gradually succumbing under the Law of Large Numbers.

The "[micro]foundations" of...models don't guarantee empirical success or policy usefulness.
What is important...is that if a model is formulated so that its parameters are economically-interpretable they will have implications for many different data sets. An aggregate theory of consumption and income movements over time should be consistent with cross-section and panel evidence...An estimate of risk aversion should fit the wide variety of situations involving uncertainty that we can observe...Estimates of labor supply should be consistent aggregate employment movements over time as well as cross-section, panel, and lifecycle evidence...This kind of cross-validation (or invalidation!) is only possible with models that have clear underlying economics: micro-foundations, if you like.
This is bread-and-butter stuff in the hard sciences.




Fighting Fiscal Phantoms, by Paul Krugman, Commentary, NY Times: These are difficult times for the deficit scolds who have dominated policy discussion for almost three years. One could almost feel sorry for them, if it weren’t for their role in diverting attention from the ongoing problem of inadequate recovery, and thereby helping to perpetuate catastrophically high unemployment.
What has changed? For one thing, the crisis they predicted keeps not happening. Far from fleeing U.S. debt, investors have continued to pile in, drivinginterest rates to historical lows. Beyond that, suddenly the clear and present danger to the American economy isn’t that we’ll fail to reduce the deficit enough; it is, instead, that we’ll reduce the deficit too much. ...
Given these realities, the deficit-scold movement has lost some of its clout. ... But the deficit scolds aren’t giving up. Now yet another organization,Fix the Debt, is campaigning for cuts to Social Security and Medicare, even while making lower tax rates a “core principle.” That last part makes no sense in terms of the group’s ostensible mission, but makes perfect sense if you look at the array of big corporations, from Goldman Sachs to the UnitedHealth Group, that are involved in the effort and would benefit from tax cuts. Hey, sacrifice is for the little people.
So should we take this latest push seriously? No... As far as I can tell, every example supposedly illustrating the dangers of debt involves either a country that, like Greece today, lacked its own currency, or a country that, like Asian economies in the 1990s, had large debts in foreign currencies. Countries with large debts in their own currency, likeFrance after World War I, have sometimes experienced big loss-of-confidence drops in the value of their currency — but nothing like the debt-induced recession we’re being told to fear.
So let’s step back for a minute, and consider what’s going on here. For years, deficit scolds have held Washington in thrall with warnings of an imminent debt crisis, even though investors, who continue to buy U.S. bonds, clearly believe that such a crisis won’t happen; economic analysis says that such a crisis can’t happen; and the historical record shows no examples bearing any resemblance to our current situation in which such a crisis actually did happen.
If you ask me, it’s time for Washington to stop worrying about this phantom menace — and to stop listening to the people who have been peddling this scare story in an attempt to get their way.
Hmmmmmn, ephemeral PK.
With the "Euro crisis" making headlines weekly, investors are indeed piling into the dollar.
Should that be dealt with, they'd be piling out of the dollar to diversify. (Not all your eggs in one basket, etc., etc., etc.)
Besides, in a climate of global economic morosity, the best investment policy is perhaps to wait it out with savings ensconced in the money market earning miserable returns but at least somewhat "safe".
N'est-ce pas vrai? (Pardon my frank-ness. ;^)
He's been doing that for the longest time. You just arrive here ... ?
MT posts a PK blog regularly and, despite comments like yours, they generally provoke a great variety of discussion in a thread.
But PK cannot publish every damn week the same post, so he seeks a bit of variety in his subjects. Get it?
Try writing a weekly column for wide syndication. Let's see how YOU write such on a weekly basis without avoiding "straw men".
Hint: It cannot be done ...
So debate the text and forget the ad-hominems.
A good place to start is a civil war for cliff certainty between red state takers and blue state makers. May those states that reach full employment first win.
If we go off that Fiscal Cliff, we're going to
scare off a lot of 'investors' who are *still*
buying out stuff (at ~zero interest) because
it's STILL such a safe investment, maybe?
Not sure how that can happen ... but I would not put anything beyond the crazies presently inhabiting LaLaLand on the Potomac.
Why would not a Golden Rule (balanced budget) be a "Great Thing" for the US, where we reduced our debt to 5/10% of GDP (from the 105% presently)? And how about if we did this with a law that lasted for ten years, that is, the time to understand how to budget expenditures under a Golden Rule.
That would force us into a "guns or butter" situation - that is US (meaning "us") or THEM (meaning the M-I-C and Finance, BigInsurance, etc.) It would also mean hiking tax rates on some people - like progressively on anyone earning beyond 10 megabucks per annum. And on anyone who possesses more than 20 megabucks a Wealth Tax of 3% of total Net Worth annually.
There is one helluva lotta people who would LIKE to earn 10 megabucks per year. I want to see just ONE justify why? (Aside from the usual, mindless refrain "Because I EARNED IT!")
MY POINT?
The annual mean wage in the US is $45,230 according to the BLS five minutes ago.
Someone please justify a wage higher than 100 times that salary (that is, 4.5 megabucks).
Their objective is to steal our Social Security. Deficits are smokescreen to hide their true intent.
Why can't pk tell us why some powerful Austerians might have good reasons
to support these budget squeezers
Get their way ?
Is it just willful ignorance or is it ...
Cui bono ?
"Beyond that, suddenly the clear and present danger to the American economy isn’t that we’ll fail to reduce the deficit enough; it is, instead, that we’ll reduce the deficit too much. ..."
OMG Kruggles is channeling Greenspan!!!! The World Is Ending!!!
Yes, I know, times change and facts change and I change my opinions.
"
deficit-scold movement
"
Concerning the assumptions of PK, what's snot to like? Do you simply love his assumptions? Have you been recently disturbed by your unfounded fears and paranoia, fears of *deficit-scald movement*? Do you now fear that federally induced national debt doubling and rapid fire redoubling will quickly put us all into the business of draining the blood of our impoverished citizens just enough to provide debt service funds to fat bankers The World Over? Doon worry! Be happy! Doon worry! Help is on the way.
The foreign banker's Dept Party Politicians have a sugar pill for you. Just take the pill now like a good lad then bright colours never seen before will dance happily before your eyes, just before you die!
Near Vanna
!
Only thing I would add to Krugman's excellent column is that Fix the Debt is not really "another" organization. It's the same old guys - Peterson, Bowles, Simpson and crew.
Negligible interest & Fiscal Cliffs not withstanding,
as long as borrowers keep borrowing & consumers
keep consuming, we must be doing great!
Keep up the good work, people!
Turkey must stimulate writing.
I would like to see a study that backs up this claim.
My guess is there are a handful first world economies that can do this but the far majority of other countries will be punished by the markets. Countries who are lucky include US, Japan and UK, although in the UK the CB buys most bonds, hardly a real market left.
Krugman than comes with the argument that in the case of France devaluation is actually not a bad thing, as devaluation grows the economy, but he doesn't mention the effect of inflating away the purchasing power of ordinary people (and again maybe the US is the exception here with reserve currency status and commodities priced in dollar).
It would be nice if Krugman would accept the special status of the USA in the world, that the US can use (and does use) to it's advantage, but I get the impression Krugman denies the special status because it doesn't serve his economic theories.
Rhetorical question, to which the answer is obviously NO. Here Krugman is referring to the theoretic "seriousness" of the confidence fairy invoking, bond vigilante fearing deficit scolds. But analytic clear headedness, as Krugman frequently laments and well knows, is not dispositive.
Answered as a political question, which is what really matters, the answer is YES. Obama appointed the catfood commission and its odious chairmen. Now "The White House has tapped the Treasury secretary as its lead negotiator in deficit-reduction talks with Congress,..." (WSJ today) Evil Uncle Timmy is more than willing to be John Alden to Obama's Miles Standish in wooing the reluctant Priscilla's support for the Grand Sellout.
"The deal is still on the table."
can you do ?
agree with PK
thank you
ps i hop is not some mistake writing ..to my coment because i relly will feel very ''bad''
I love the classics, they never go out of style.
I'm pretty sure you don't know how to debate science, but it needs to be done with published papers--not blog posts.
Mulligan has a peer-reviewed, published paper (and book). In rebuttal, you have cut and pasted snippets from liberal economist's blogs.
Just so you know, this is NOT debating science.
Think for yourself; get on Google Scholar (use your fav econo guys as a starting point), and find me a legitimate govt or scholarly paper that explains why marginal tax rates DON'T impact workforce participation, especially for those near the poverty level. I'd LOVE to read it.
THIS is debating science.
It's hard to publish a scientific paper; blog posts on the other hand are a dime a dozen.
I'll start again (we'll see if you get the dance). Here's the CBO explaining marginal tax rates for those across the lower income spectrum. Do you see those high rates for the poor?
http://www.cbo.gov/sites/default/files/cbofiles/attachments/11-15-2012-MarginalTaxRates.pdf
Per the CBO (and Mulligan), the poor face extremely high marginal tax rates. These rates accelerate from 10 to 30-plus percent as earnings move from 0 percent of poverty, to 150% of poverty.
This is a extreme disincentive for the poor to enter the workforce.
Now it's your turn. NO cut and paste. Do REAL work--you might stumble across something to feed your values.
I'd better stick with the published literature, just to keep my license in-tact.
And I did provide a scholarly paper (and a CBO report to back up my point). You provided toilet paper.
Bye for now.
This is a definite disincentive and should be fixed. There should be a marginal reduction of food stamps as you go over above the 1741 number and which reduces to 0 at a point you are earning well above poverty levels.
And no, this disincentive did not cause the recession. Thats a pretty stupid, romney like conclusion.